How do we use mathematical shorthand to write very large and very small numbers? We focus on the most important digits, and use an abbreviation to avoid writing out the insignificant digits.
Avogadro number 6.022 x 10 23 23 atoms per mole. Answer to one decimal places. Units in scientific notation as in 10 X. The Avogadro's number is equal to (6,022 x 10 raised to 23 particles) and is symbolized in the formulas with the letters L or NA. In addition, it is used to make conversions between grams and atomic mass unit. The unit of measure of the Avogadro's number is the mole (mol-1) but it can also be defined in lb/mol-1 and oz/mol-1.
Usually the only significant information is in the first couple of digits and the location in place value that they occupy.
Scientific Notation
- Allows us to compactly write very large or very small numbers
- Creates a shorthand that allows us to simplify calculation and avoid errors
- Note that you already use scientific notation when you speak about large numbers
- You don’t say one zero zero zero zero zero zero
- You say one million
Orders of magnitude
- Powers of ten
- Place value representation
- Operating on numbers with scientific notation
Representing large numbers
There are different ways to express scientific notation
- On paper 1.23 times 10^4
- On a calculator 1.23 (EE key) 4
- On a computer
1.23E4
A very large number
- Avogadro’s Number
- 6.02 times 10^{23}
- 10^{1} = 10
- 10^{2} = 10 times 10
- 10^{3} = 10 times 10 times 10
A very small number
- Gravitational Constant
- 6.67 times 10^{-11} (m^3 kg^{-1} s^{-2})
- 10^{-1} = frac{1}{10}
- 10^{-2} = frac{1}{10 times 10}
- 10^{-3} = frac{1}{10 times 10 times 10}
Operations
- Addition
- Subtraction
- Multiplication
- Division
Multiplying and dividing large numbers
10^a cdot 10^b = 10^{a+b}
c cdot 10^a cdot d cdot 10^b = c cdot d cdot 10^{a+b}
frac{10^a}{10^b} = 10^{a-b}
frac{c cdot 10^a}{d cdot 10^b} = frac{c}{d} cdot 10^{a-b}
You can combine these rules if you are multiplying and dividing several numbers in scientific notation.
Help With Scientific Notation
Common Powers
Notice that these prefixes correspond to english language quantities you are already familiar with. You often express large numbers verbally, so there isn’t much new to learn to use metric prefixes or scientific notation.
For example, 1 billion joules is the same as a gigajoule is the same as 1 cdot 10^{9} joules is the same as 1,000,000,000 joules.
A more complete list can be found here: Wikipedia Metric Prefixes
| greek prefix | english name | exponent |
|---|---|---|
| kilo | thousand | 10^3 |
| mega | million | 10^6 |
| giga | billion | 10^9 |
| tera | trillion | 10^{12} |
| peta | quadrillion | 10^{15} |
| exa | quintillion | 10^{18} |
Learning Objective
Learn to express numbers in scientific notation.
Throughout this course, you will frequently encounter very large and very small numbers. Handling these numbers can be quiet cumbersome, especially when performing calculations.
For example, later on in this course we will be working with Avogadro’s number (the number of elementary entities in 1 mole of a substance).
Avogadro’s number: 602,200,000,000,000,000,000,000
Avogadro’s number, as show above, is expressed in standard notation. For relatively small numbers, standard notation is fine. However, for very large numbers, such as Avogadro’s number, or for very small numbers, such as 0.000000419, using standard notation can be cumbersome because of the number of zeros needed to place nonzero numbers in the proper position. In order to make it easy to handle large or small numbers scientist use a method called scientific notation. Regardless of the magnitude, all numbers can be expressed in the form of scientific notation, N × 10n, where N is a number between 1 and 9, and n is either a positive or negative whole number.
Avogadro’s number in scientific notation form: 6.022 × 1023
Scientific notation is an expression of a number using powers of 10. Powers of 10 are used to express numbers that have many zeros:
| [latex]large 10^{0}=1[/latex] |
| [latex]large 10^{1}=10[/latex] |
| [latex]large 10^{2}=10times10=100[/latex] |
| [latex]large 10^{3}=10times10times10=1000[/latex] |
| [latex]large 10^{3}=10times10times10times10=10000[/latex] |
The raised number to the right of the 10 indicating the number of factors of 10 in the original number is the exponent. (Scientific notation is sometimes called exponential notation.) The exponent’s value is equal to the number of zeros in the number expressed in standard notation.
Small numbers can also be expressed in scientific notation but with negative exponents:
| [latex]large 10^{-1}=frac{1}{10}=0.1[/latex] |
| [latex]large 10^{-2}=frac{1}{100}=0.01[/latex] |
| [latex]large 10^{-3}=frac{1}{1000}=0.001[/latex] |
| [latex]large 10^{-4}=frac{1}{10000}=0.0001[/latex] |
Again, the value of the exponent is equal to the number of zeros in the denominator of the associated fraction. A negative exponent implies a decimal number less than one.
A number is expressed in scientific notation by writing the first nonzero digit, then a decimal point, and then the rest of the digits. The part of a number in scientific notation that is multiplied by a power of 10 is called the coefficient. Then determine the power of 10 needed to make that number into the original number and multiply the written number by the proper power of 10. For example, to write 79,345 in scientific notation,
79,345 = 7.9345 × 10,000 = 7.9345 × 104
Thus, the number in scientific notation is 7.9345 × 104. For small numbers, the same process is used, but the exponent for the power of 10 is negative:
[latex]large 0.000411 = 4.11times frac{1}{10000} = 4.11times 10^{-4}[/latex]
Typically, the extra zero digits at the end or the beginning of a number are not included.
Another way to determine the power of 10 in scientific notation is to count the number of places you need to move the decimal point to get a numerical value between 1 and 10. The number of places equals the power of 10. This number is positive if you move the decimal point to the right and negative if you move the decimal point to the left.
For example, Express the following number in scientific form: 3,500 and 0.000054.
3,500, move the decimal point to the left three spots to give 3.5 × 104
0.000054, move the decimal point to the right five spots to give 5.4× 10-5
Example 1: Writing Numbers in scientific notation
Express these numbers in scientific notation.
- 306,000
- 0.00884
- 2,760,000
- 0.000000559
- 23,070
- 0.0009706
 Show Answer
Show Answer- The number 306,000 is 3.06 times 100,000, or 3.06 times 105. In scientific notation, the number is 3.06 × 105.
- The number 0.00884 is 8.84 times 1/1,000, which is 8.84 times 10−3. In scientific notation, the number is 8.84 × 10−3.
- The number 2,760,000 is 2.76 times 1,000,000, which is the same as 2.76 times 106. In scientific notation, the number is written as 2.76 × 106. Note that we omit the zeros at the end of the original number.
- The number 0.000000559 is 5.59 times 1/10,000,000, which is 5.59 times 10−7. In scientific notation, the number is written as 5.59 × 10−7.
- The number 23,070 is 2.307 times 10,000, or 2.307 times 104. In scientific notation, the number is 2.307 × 104.
- The number 0.0009706 is 9.706 times 1/10,000, or 9.706 times 10-4. In scientific notation, the number is 9.706 × 10-4.
Many quantities in chemistry are expressed in scientific notation. When performing calculations, you may have to enter a number in scientific notation into a calculator. Be sure you know how to correctly enter a number in scientific notation into your calculator. Different models of calculators require different actions for properly entering scientific notation. If in doubt, consult your instructor immediately.
Key Takeaways
- Standard notation expresses a number normally.
- Scientific notation expresses a number as a coefficient times a power of 10.
- The power of 10 is positive for numbers greater than 1 and negative for numbers between 0 and 1.
This calculator shows only the coefficient and the power of 10 to represent the number in scientific notation. Thus, the number being displayed is 3.84951 × 1018, or 3,849,510,000,000,000,000.
Source: “Casio”Asim Bijarani is licensed under Creative Commons Attribution 2.0 Generic
Exercises
a) 56.9
b) 563,100
c) 0.0804
d) 0.00000667
2. Express these numbers in scientific notation.
a) −890,000
b) 602,000,000,000
c) 0.0000004099
d) 0.000000000000011
Avogadro's Number In Scientific Notation 0 0021
3. Express these numbers in scientific notation.
a) 0.00656
b) 65,600
c) 4,567,000
d) 0.000005507
4. Express these numbers in scientific notation.
a) 65
b) −321.09
c) 0.000077099
d) 0.000000000218
5. Express these numbers in standard notation.
a) 1.381 × 105
b) 5.22 × 10−7
c) 9.998 × 104
6. Express these numbers in standard notation.
a) 7.11 × 10−2
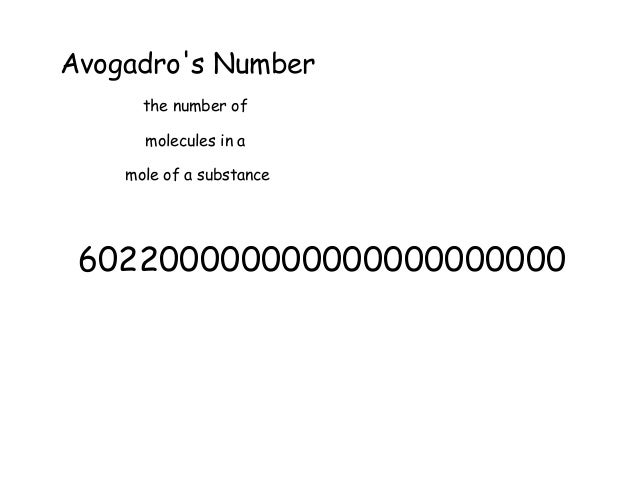
b) 9.18 × 102
c) 3.09 × 10−10
7. Express these numbers in standard notation.
a) 8.09 × 100
b) 3.088 × 10−5
c) −4.239 × 102
8. Express these numbers in standard notation.
a) 2.87 × 10−8
b) 1.78 × 1011
c) 1.381 × 10−23
9. These numbers are not written in proper scientific notation. Rewrite them so that they are in proper scientific notation.
a) 72.44 × 103
b) 9,943 × 10−5
c) 588,399 × 102
10. These numbers are not written in proper scientific notation. Rewrite them so that they are in proper scientific notation.
a) 0.000077 × 10−7
b) 0.000111 × 108
c) 602,000 × 1018
11. These numbers are not written in proper scientific notation. Rewrite them so that they are in proper scientific notation.

a) 345.1 × 102
b) 0.234 × 10−3
c) 1,800 × 10−2
12. These numbers are not written in proper scientific notation. Rewrite them so that they are in proper scientific notation.
a) 8,099 × 10−8
b) 34.5 × 100
c) 0.000332 × 104
13. Write these numbers in scientific notation by counting the number of places the decimal point is moved.
a) 123,456.78
b) 98,490
c) 0.000000445
14. Write these numbers in scientific notation by counting the number of places the decimal point is moved.
a) 0.000552
b) 1,987
c) 0.00000000887
15. Use your calculator to evaluate these expressions. Express the final answer in proper scientific notation.
a) 456 × (7.4 × 108) = ?
b) (3.02 × 105) ÷ (9.04 × 1015) = ?
c) 0.0044 × 0.000833 = ?
16. Use your calculator to evaluate these expressions. Express the final answer in proper scientific notation.
a) 98,000 × 23,000 = ?
b) 98,000 ÷ 23,000 = ?
c) (4.6 × 10−5) × (2.09 × 103) = ?
17. Use your calculator to evaluate these expressions. Express the final answer in proper scientific notation.
a) 45 × 132 ÷ 882 = ?
b) [(6.37 × 104) × (8.44 × 10−4)] ÷ (3.2209 × 1015) = ?
18. Use your calculator to evaluate these expressions. Express the final answer in proper scientific notation.
a) (9.09 × 108) ÷ [(6.33 × 109) × (4.066 × 10−7)] = ?
b) 9,345 × 34.866 ÷ 0.00665 = ?
Show Answer to Select Questions1.
a) 5.69 × 101
b) 5.631 × 105
c) 8.04 × 10−2
d) 6.67 × 10−6
3.
a) 6.56 × 10−3
b) 6.56 × 104
c) 4.567 × 106
d) 5.507 × 10−6
5.
a) 138,100
b) 0.000000522
c) 99,980
7.
a) 8.09
b) 0.00003088
c) −423.9
9.
a) 7.244 × 104
b) 9.943 × 10−2
c) 5.88399 × 107
Avogadro's Number Chemistry Definition
11.
a) 3.451 × 104
b) 2.34 × 10−4
c) 1.8 × 101
13.
a) 1.2345678 × 105
b) 9.849 × 104
c) 4.45 × 10−7
15.
a) 3.3744 × 1011
b) 3.3407 × 10−11
c) 3.665 × 10−6
Convert To A Scientific Notation
17.
a) 6.7346 × 100
b) 1.6691 × 10−14
